Measure the Earth Experiment

[22^nd December, 2007]

How big is the Earth?

The impact of this question and the answer is always unclear to school children. Although they are taught in Geography class, all they get is some printed data like radius = 6400km. We also do not relate this important question to applied mathematics. Geometry is a whole branch that came out of measuring the Earth! To make this process apparent and easier for teachers, the following project has been undertaken by IUCAA and Vigyan Prasar

Eratosthenes & his method

Eratosthenes, the Greek mathematician, poet, athlete, geographer and astronomer, is credited for the first estimation of the size of the Earth around 240 BC. He was the librarian of the great library of Alexandria in Egypt. Although he could read the latest scientific knowledge of his day, he was always interested in learning new things. Being without much equipment and technology, he used only tools like sticks, eyes, feet and brains to do this experiment.

It was not such a simple thing those days to believe that the Earth was round! Most people thought it was flat. But Eratosthenes went ahead to measure the sphere he believed the Earth to be. But, he did not use methods like walking around the Earth or digging a hole through its centre to the other side.

The Earth, if cut into two halves, would show a circular section. The boundary of the circle would be its circumference, which we want to measure. Suppose this circle was a Cake. We 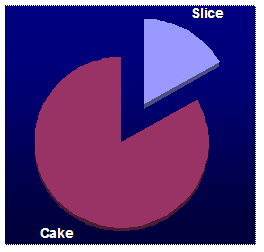cut out a slice that is one-sixth [1/6] of it. The curved edge of this will then be 1/6^th of the circumference. To put it the other way, the circumference of the cake will be six times the length of the curved edge of the slice. If we measure the angle made by the other two sides, then we would find that it is 60^o. It has to be so, because 60^o is 1/6^th of 360^o which is the whole circle. Thus if we know the distance between two points on a circumference and know the angle made by them at the centre, we can find out the length of the circle. This means that if we know the distance between two points on the Earth and the angle they make with the Earth’s centre, we can find out the circumference of the Earth.

The distance between two places can be measured. The problem is to measure the angle that they make. This was solved by Eratosthenes.

He had heard that in a place called Syene, south of Alexandria, the walls of a well cast no shadow in the water at noon on the day of the middle of Summer. In other words, there were no shadows of by vertical things June 21^st, as the Sun was exactly overhead there at noon.

But on the same date he observed that a vertical pillar in Alexandria cast a small but measurable length of shadow. If the Earth was flat, then the sunrays coming perpendicular to the flatland would make all vertical objects cast similar shadows anywhere on the Earth. The difference in the length is possible only if the Sunlight comes at different angles at different places. This means that the Earth is a curved surface.

If two sticks perpendicular to the ground in Syene and Alexandria were to extend to the centre of the Earth, they would thus make an angle. Using simple geometry, he found out this angle from the length of the shadow at Alexandria on 21^st June. It came out to be about 7^o which is 1/50^th of 360^o. It is said that he got an army to walk to Syene [now called Aswan] to measure the distance till there. It came out to be 800km. Fifty times that i.e. 40,000km was the circumference of the Earth!

Aim

The aim of this activity is to get teachers and students to measure the dimensions of the Earth using the same simple method used by Eratosthenes over 2000 years ago. Participants are to make a simple setup and measure some shadows and note some times. If s & q are known in the figure below, we can find the circumference of the Earth.

To get the value of the Earth's circumference, Eratosthenes had to measure the distance between the two places that he did the experiment at. But now that we know the circumference very accurately, we can reverse the method and use it to find the latitude and longitude of the place where our observations are taken.

More...

The scope of this project to teach applied geometry and trigonometry is great. The radius and other parameters of a sphere can be found out by the children using the circumference. This project, if done in a group across the country, can also help you verify your latitude and your longitude. A comparison with other groups and some calculations can give us the easily give us these values. If two places collaborate, they can also find out the distance between them.

Instead of doing it on just one day, this can also be repeated periodically for years to come so that a new group of students get to learn form this exercise every year.

How you and your group may participate in the project

With your group participating along with the others all over the country, we will be able to repeat this fundamental measurement by Eratosthenes. We give here some basic instructions for you to follow. If you have understood the Eratosthenes method you may modify the method given here to suit your local conditions. However, it is advisable that all of us to follow these instructions for the uniformity and to cross check the observations.

In this experiment we will be measuring a shadow of a tall pole due to the sunlight, for about 4 hours from 10:00 a.m. to 2 p.m.

What you have to do

Requirements and Preparations

You should find a place where you can get a clear sunshine from 10am till about 2:30pm. Your cricket or football ground should be quite good.
We need a cylindrical pole of 25 mm in diameter and about 200 cm long. The pole could be 220 to 240 cm too as long as only 200 cm of it used for casting the shadow.
Measuring tape. This will be used to measure the length of the shadow of the pole.
An accurate clock.
Big marbles or small white painted stones or big nails (~30 in number), chalk etc.
Plumb-line

Prior to the actual experiment

Three to five days before the actual date of the experiment locate the place where you would like to do the experiment and fix the pole and watch the shadow of the pole. Check if it gets the full sunshine from 10 am to 2 p.m. It can and might happen that at a crucial time shadow of a nearby building might fall on the pole spoiling your experiment. Shift the position of the pole if necessary.

The pole is to be rooted in the ground firmly. It is important, for this group project, that it is exactly 200 cm above the ground. For this, measure 200cm length from one end of the pole and mark this point with coloured tape. Bury the pole such that the portion after this goes inside and the tape is just touching the ground.

Now if you are fixing the pole in soil then you should dig a deep hole and put the base into it such that 200 cm stick out above the ground. Pack it with small stones and pebbles and then add loose soil. Make the pole exactly vertical with the help of a plumb-line. Put water to fix it.

If you school has tiled floor or if you would like to do the experiment on the terrace of your school then you may make a stand to support the pole but remember that the place of the pole should not be disturbed during the entire experiment. Again use the plumb-line to make the pole vertical.

You have to check your watch if it is accurate. Tune in the All India Radio (AIR) just before the morning news at 8 a.m. Prior to the start of the News you will hear beep. When you hear the long beep it mean it is exactly 8 a.m. Check time in your watch matches with that of AIR if it does then it is ok of not correct the watch and check it the next day again. If there is a problem then either get a better watch or correct your time. The clocks in Mobile phones are quite accurate.

On the day of the experiment

1. Be at the observing station i.e. where the pole is anchored, by about 9:30 a.m. and get ready to start the observations by filling up the form given here.

2. Now exactly at 10 a.m. mark the shadow of the pole. If you are doing the experiment in the field – place one of the marbles or stone or hammer a nail etc. in the ground. If you are on the terrace or on the tiled floor then mark the tip of the shadow with chalk.

3. Continue the observations every 10 minutes.

4. But don’t worry if you missed one or two observations because of clouds or some other disturbances. Do not put marks on your own to make up for it!

Using the observations

The first inspection of the observations should show you when the shadow was shortest and at what time. At this time the shadow was exactly North-South. – Can you tell us why?

Plot your observations on the graph paper and draw a smooth curve. What we mean is that you are not expected to join the points. There might be some points above and some below this curve. This curve is parabolic. An example is shown on the next page.

The lowest point (or vertex of the parabola) gives us the minimum length of the shadow, h and the time at which it was minimum t.

Calculations

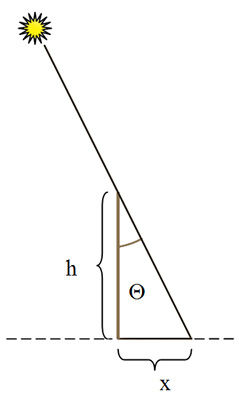
Make a scale drawing of your pole and least shadow at right angles to each other. Complete the triangle and measure the angle on top with a protractor. We denote this number by Q. One can also use trigonometry to find it.

The graph above is the data plotted using the observations

This graph is "trendline" drawn using second order polynomial

How to find out...
[Specific to 22^nd December 2007]

Latitude of the place

On this the latitude of your station would be given by Q - 23.5 deg. (the declination of the sun would be 23.5 deg. Please refer to celestial coordinate system)

Here h is the height of the vertical pole and x is the length of the shadow. You can find the angle Q making a scale drawing on a graph sheet and measuring the angle or by using trigonometric fun ‘tan’.

tan Q = x/h or Q = tan^-(x/h)

Longitude of the place :

Longitude of the place would be the time difference between the time at which the shadow was minimum at you place and the time at which the shadow was minimum at a place whose longitude we know. See the table below

	Latitude (N)	Longitude (E)
Vigyan Prasar, Noida	28^o 37’	77^o 15’	5h 09m
IUCAA – MVS, Pune	18^o 33’	73^o 49’	4h 55m

Example: On 22^nd Dec 07 at Vigyan Prasar, Noida and IUCAA the shadow would be shortest at 12:19 and 12:33 respectively. This time is also called transit time of the sun. Now say at your station the time at which the shadow was minimum was at 12:21. Which is 2 min after the transit time at Vigyan Prasar or 12 min before the transit time at IUCAA Pune.

[All times are in IST]

Therefore the longitude of your place would be

[5h 09m – 0h 2m] = 5h 07m or [4h 55m + 0h 12m] = 5h 7m or 76^o 45’.

Measure the Earth experiment

Observation Log

Name and address of the Organization (school, amateur astronomer's clubs etc)

------------------------------------------------------------------------------------------------------------

Name of the principle investigator(s) (PI)

------------------------------------------------------------------------------------------------------------

Height of the vertical pole . . . . . . . . . . . . . . . . . .

(Even though we have fixed the length of the pole to be exactly 200 cm above the ground but it would pay to check it out and if it is not then write the exact length.)

Observation table (write length in centimeters)

Time in (IST)	Length of the shadow	Time in (IST)	Length of the shadow	Time in (IST)	Length of the shadow	Time in (IST)	Length of the shadow
10:00		11:00		12:00		13:00
10:10		11:10		12:10		13:10
10:20		11:20		12:20		13:20
10:30		11:30		12:30		13:30
10:40		11:40		12:40		13:40
10:50		11:50		12:50		13:50

Names of the other participants

------------------------------------------------------------------------------------------------------------

Measure the Earth experiment

Calculations and results

x ......................... t .........................

Q ............................ (it was obtained by - graphical method / using calculator, tick one)

Latitude of the place (Q - 23.5 deg)...........................................

Longitude of the place

Time at which the shadow was minimum at

Vigyan Prasar, Noida ............... - ............... = .....................

(VP time) (your time) (time difference)

Longitude at your place 5h 09m - ..................... = ....................

(VP longitude) (time difference) (your longitude in time)

or ....................... x 15 = .................. your longitude in degrees

(your long. in time)

Time at which the shadow was minimum at

IUCAA MVS, Pune .................. - ............... = ......................

(IUCAA time) (your time) (time difference)

Longitude at your place 4h 55m - ..................... = ....................

(IUCAA longitude) (time difference) (your longitude in time)

or .................... x 15 = .................. your longitude in degrees

(your long. in time )

Doing the experiment

1) One can carry out the experiment (almost) single handed. All one needs to do is to mark the position of the mast. (Here an adjustable mast is being used.)

2) One would need some help in measuring the length of the shadow.

3) The floor on which this experiment was carried out has the "graph lines" made during the cementing of the floor. One can, therefore, measure "x" and "y" and calculate the shadow length.

4) But it could be fun doing in a group helping each other.

We thank Neha (1st year BSc, Fergusson college), Madhuvanti and Mayuri (X std, DAV Public School), Dhruv and Rohan (VI std, Loyola School) And Jyoti Hiremath for helping out Neha.

Extra Information about Eratosthenes:

Eratosthenes was born in Cyrene (in modern-day Libya), he worked at Alexandria and died in capital of Ptolemaic Egypt. He was never married. In 236 BC he was appointed by Ptolemy III Euergetes I as librarian of the Alexandrian library. He made several important contributions to mathematics and science, and was a good friend to Archimedes. Around 255 BC he invented the armillary sphere, which was widely used until the invention of the Orrery in the 18th century. In 194 BC Eratosthenes became blind and a year later he supposedly starved himself to death.

Although Eratosthenes' method was well founded, the accuracy of his calculation was inherently limited. The accuracy of Eratosthenes' measurement would have been reduced by the fact that Syene is not precisely on the Tropic of Cancer, is not directly south of Alexandria, and the Sun appears as a disk located at a finite distance from the Earth instead of as a point source of light at an infinite distance. To put concisely

The two cities are not on the same meridian (the difference in longitude is 3^o);
Syene is not on the Tropic of Cancer (it is situated 55 km North);
The angular difference is not 7^o 12' but 7^o 5'.
The actual distance between Alexandria and Syene is 729 km, not 800km.

There are other sources of experimental error: the greatest limitation to Eratosthenes' method was that, in antiquity, overland distance measurements were not reliable, especially for travel along the non-linear Nile which was traveled primarily by boat. For overland distance measurement, the accuracy rests on the estimated average speed of a caravan of camels. So, the accuracy of Eratosthenes' size of the earth is surprising.

Eratosthenes' experiment was highly regarded at the time, and his estimate of the Earth’s size was accepted for hundreds of years afterwards. His method was used by Posidonius about 150 years later.

Weblinks

Other Eratosthenes’ experiment projects:

http://www.k12science.org/noonday/

http://www.physics2005.org/projects/eratosthenes/experiment.html

http://www.algonet.se/~sirius/eaae/aol/market/collabor/erathost/

http://www.phys-astro.sonoma.edu/observatory/eratosthenes/

http://www.youth.net/eratosthenes/

About Eratosthenes:

http://en.wikipedia.org/wiki/Eratosthenes

Contact

Mr. Arvind Paranjpye OR Mr. Samir Dhurde

(020-25604601) (020-25604603)

(arp@iucaa.ernet.in) (samir@iucaa.ernet.in)

PO Bag #4, IUCAA,

Ganeshkhind, Pune -411007

OR Vigyan Prasar